Issues in loudspeaker design - 1 

| Page 1 | Page 2 | Page 3 | Page 4 | Page 5 | Page 6 | Page 7 |

-----------------------------------------------------------------------------------------------------------------------------------------------------------------

Introduction & Content

When it comes to the practical requirements for accurate sound reproduction, then there are a surprising number of issues that are not fully understood. Sound reproduction is about creating illusions in our mind, in that amazing information processor between our two ears. Sound reproduction involves physical processes to generate air vibrations that move our eardrums and in turn stimulate mental processes, that have evolved over millions of years for the purpose of survival and for communication, processes that lead to perception of the sound source and its environment. While we can measure many different physical parameters involved in the generation and propagation of a sound field, it becomes exceedingly difficult to assess their relative importance to what we hear and how it helps or detracts from the illusion. The published scientific material on the psychology of hearing (psychoacoustics) is extensive and sometimes helpful in explaining phenomena in two-channel and surround sound reproduction. 

In the following I describe what I see as frontiers, if not in yours, then at least in my understanding of what matters for accurate sound reproduction.  While I strive as an engineer to find answers and explanations based on the physics of the situation, I try to be honest to my listening observations as ultimate arbiter. I use my memory of un-amplified sound as reference for judging accuracy of the illusion. What I see as frontiers may be settled areas to others, or clear separations between opposing camps, that hold on to cherished convictions. I would hope that the exposé stimulates a few readers to research a subject further and share their insights.

A - Acoustic absorption and acoustic resistors

B - Frequency response of open baffles 

C - Dipoles below the Schroeder frequency of a room

D - Room and baffle reflections

E - Line sources

F - Group delay and transient response

G - Multitone distortion

H - Suggestions and hearing

I - Digital crossovers

J - Doppler distortion

K - Room response equalization

L - H-frame and U-frame open-baffle woofers

M - Investigation of energy storage

N - Mounting a driver to a baffle

O - Unreasonably high distortion in midrange drivers?

P - Estimate of L_e(x) induced distortion at high frequencies

Q - Estimate of L_e(i) induced distortion at high frequencies

R - Recommended study material about distortion in loudspeaker drivers

S - Dipole and monopole woofer response

T - Separate woofer crossover and offset

U - Woofer level setting

V - Crossover topology issues

W - Accurate performance of a stereo sound system

X - Spatial distortion in audio

Y - Triggered burst measurements of tweeters

Z - Accuracy, spatial distortion and plausibility of the auditory scene

A2 - High -frequency down-shelving for ORION-3

B2 - What is the optimum polar response for a loudspeaker?

C2 - L-07 Dipole loudspeaker

D2 - Phase shift due to dipole D and its effect upon crossovers

E2 - Sound field control

F2 - Woofer equalization in LXmini

G2 - Power amplifier distortion at 1 W & 100 mW output

--------------------------------------------------------------------------

A - Acoustic absorption and acoustic resistors

When a speaker driver is mounted in a box it radiates as much energy into the space in front of the cone as it does into the much smaller space behind the cone. What happens to the air borne energy inside? At long wavelengths it is common practice to store it in resonant structures to extend the steady-state low frequency response of the speaker. In general, the energy leads to very high sound pressures inside the box.  A small amount of the energy is lost as heat in the stuffing material, some in the process of flexing the cabinet walls. Much of it reappears outside the box, because the thin cone presents a weak sound barrier. Just how much is difficult to measure, but it is a contributor to the frequency response. I am of the opinion that the effect is most notable in the low hundreds of Hz region, where stuffing materials are ineffective and the internal dimensions not small enough for the internal air volume to act as a pure compliance. Consequently, enclosures should be either very small (less than 1/16th of a wavelength) or extremely large, both of which are not very practical for different reasons. 

To make progress with box speakers an acoustic resistor is needed that can more effectively dissipate energy in the 80 Hz to 800 Hz frequency range at high volume velocities. Such device would not only be useful for closed box speakers, but also for speakers that use the rear radiation from the driver to form a specific polar radiation pattern, such as a cardioid.
A cardioid speaker can be made with two opposite polarity monopole sources separated by a distance D, and with the signal to one of the sources delayed by a time T = D/c. An implementation of this concept could be a driver in a box of depth D where the rear wall is an acoustic resistor R. At long wavelengths the box internal air volume behaves as a compliance or acoustic capacitor C. The acoustic output from the rear of the box is low-passed by the RC filter and delayed relative to the front output by T = RC.

The acoustic resistor should be purely dissipative, with vanishing reactive component, and be independent of frequency. It also should be linear over the range of volume velocities encountered for high SPL. Traditionally cloth type materials have been used for cardioid speakers. Long fiber wool, synthetic fibers or fiber glass matting  have been used to attenuate sound inside enclosures. The properties of these materials are neither frequency independent nor linear. 

It may not be widely known that filter media for the filtration of liquids and gases in the chemical and other industries can have applications in acoustics. Such filters may be thin sheets (<1 mm thick) of a non-woven, sintered, stainless steel fibre matrix for filtration levels from 5 to 50 micron. Airflow at a constant velocity v through the filter material causes a pressure drop Dp between input and output sides corresponding to a flow resistance R_f =  Dp/v [Ns/m³]. 
It is common in this industry to specify an inverse quantity which is Permeability P [l/dm³/min] at 200 Pa pressure drop. Flow resistance and permeability are related by R_f = 1200/P in this case. 
Resistance values between 150 and 3500 Ns/m³, or 15 to 350 rayl in the older cgs system of units (1 rayl = 10 Ns/m³), are obtainable from a single filter sheet. For comparison the free-space acoustic field impedance p/v = rc  is resistive and has a value of 414 Ns/m³ = 41.4 rayl.
Materials are available with greater structural rigidity such as Feltmetal with thickness up to 6 mm and resistance between 6 and 50 rayl. The impedance is resistive and constant over the 20 Hz to 2 kHz range that I tested. Linearity should also be quite good, but I have not measured it.

The challenge remains to build an acoustic termination for the inside of a box. Feltmetal and filters should be readily useable for a cardioid speaker, but for a woofer application their linearity at high volume velocities needs investigation.
Top

B - Frequency response of open baffles

At this point in time the design of an open baffle speaker using cone type drivers is highly empirical. The baffle shape which determines the on-axis and off-axis frequency response must be optimized by experiment. This is how the dimensions of the PHOENIX main panel were determined (Ref.2), because no modeling software was available. Some guidance for baffle size can be derived from the response of a flat, thin circular disk baffle of radius D with the driver in its center. This shape is less than optimum for use at frequencies above f = 0.5 c/D, where comb filtering occurs. The peaks and dips in response are smoothed by a flat rectangular baffle. As the driver itself becomes more directional the outline of the baffle has less effect.

For size, appearance and structural reasons the baffle may be folded back into a shallow U-shape. At low frequencies the H-shape and W-shape baffles lead to space savings. All of theses structures are considerably more difficult to analyze, because lumped and distributed acoustic elements have been added in the process of folding.

The radiation of the driver itself to front and rear is not symmetrical. The basket structure adds a complicated acoustic lowpass filter. This affects not only the output to the rear of an open baffle, but also the response in front due to additional phase shift and change in amplitude.

While it is not too difficult to estimate the response of H and W baffle structures at long wavelengths, it would be highly desirable to have modeling software for flat and U-shaped baffles for the whole frequency range. To be of usefulness the software would have to account for the measured frequency response of the driver in an 'infinite' size baffle, for radiation in front and rear and at different angles.
Top

C - Dipoles below the Schroeder frequency of a room

The Schroeder frequency describes the approximate border between the frequency range of discrete, sparse room mode distribution below it and high mode density above it. 
           f_s = 2000 ( T₆₀ / V )^1/2     [Hz, s, m³] 
For typical domestic listening rooms f_s is in the order of 100 - 200 Hz. 

Above f_s a dipole source is in the reverberant field and has the following behavior:

• 1/3rd or -4.8 dB of the radiated acoustic power compared to a monopole for the same direct sound pressure level, due to the cos(angle) free-space polar pattern.
• A 4.8 dB higher level of direct to reverberant sound compared to a monopole.
• Rotation of the source affects the direct sound level, but this is strongly masked, because of the typically higher reverberant sound level. 
• Room placement and rotation have little practical effect upon the reverberant sound field level. The number of possible modes is so high, that reducing the excitation of some increases it for others. 
• Reflections are affected by placement and rotation.

Below f_s in the sparse mode region:

• Fewer modes are excited compared to a monopole, because of the cos(angle) directivity.
• A monopole and a dipole source in the same location will excite a given mode to a different degree, because their source impedances are very different. Consequently, the match to the acoustic field impedance of the mode is different.
• Monopole and dipole source in the same location will excite a given mode to a different degree for an additional reason. For maximum excitation by a dipole source the direction of mode propagation has to coincide with the free-space direction of maximum source radiation. 

The observed audible difference between monopole and dipole for bass below f_s is:

• Less boom and droning of specific notes with a dipole,
• A higher degree of articulation and resolution of complex musical bass lines,
• A sense of air, spaciousness, and very natural reproduction of acoustic bass,
• Less physical impact,
• Less absolute bottom foundation below the lowest room mode frequency.

To my knowledge these and similar subjective observations have not been explained satisfactorily by theory and only partially by measurement. In Ref.1 I have investigated dipole behavior on the basis of:

• Steady-state frequency response of the transfer function between source and listener for a simple 2-dimensional model and measurements in a very small office room and a living room,
• Cumulative spectral decay measurements,
• Energy-time curve of the low frequency impulse response,
• Modulation transfer function for low frequencies,
• Frequency response to shaped tonebursts, 
• Frequency and time response to a multi-burst signal and its correlation to listening impression.

The best physical evidence for dipole benefits, that I have found, comes from multi-burst tests. A CD with such test signals is available. 
The steady-state frequency response has been used for arguments pro and con dipole low frequency sources. While the transfer functions for dipole and monopole are clearly different I would not dare to make sound quality judgments based either on the data that I have seen or the ones I have taken myself.  The exception to this is the very low frequency region, below the first mode, where the monopole response gains consistently from pressure buildup compared to a dipole. 

I suspect that the perceived low frequency benefits of dipoles are related to the psychology of hearing and involve much more complex processes than a simple steady-state response analysis. Our mind is geared towards analyzing time, frequency and amplitude variant sound pressure between two ears and selectively draws information from it.

My hypothesis is that three effects contribute to the subjectively more accurate reproduction of bass:
1 - The open baffle, dipole woofer has a figure-of-eight radiation pattern and therefore excites fewer room modes.
2 - Its total radiated power is 4.8 dB less than that of a monopole woofer for the same on-axis SPL. Thus the strength of the excited modes is less. 
3 - A 4.8 dB difference in SPL at low frequencies is quite significant, due to the bunching of the equal loudness contours at low frequencies, and corresponds to a 10 dB difference in loudness at 1 kHz.
Thus, bass reproduced by a dipole would be less masked by the room, since a dipole excites fewer modes, and to a lesser degree, and since the perceived difference between direct sound and room contribution is magnified by a psychoacoustic effect. 
Top

D - Room and baffle reflections

Reflections are part of everyday life. If you have been inside an anechoic chamber and experienced the eerie silence and feeling of disorientation, then you know what I mean. In more common surroundings, every sound we make generates a set of reflections. If the sound is sustained, then its amplitude and phase at a given location are affected by the surroundings. This is what happens in a listening room. To quote: 
"The acoustics of a room resemble either a linear filter or a hall of mirrors, depending upon the time scale of interest. As a filter, a room causes linear distortion in the amplitudes and phases of steady-state tones received by the listener's ears. As a hall of mirrors, a room appears to be a collection of spatially distributed reflecting surfaces that create multiple versions of each transient sound. The reflected transients ultimately reach the listener from diverse directions, almost none of which agree with the direction of the source. Thus, both the steady-state and the transient response of a room result in the listener receiving misleading cues about the location of a source of sound in the room." [1]

Modeling and understanding of how we manage to cope with this situation, and actually localize real and virtual sources surprisingly well in most instances, is subject of ongoing scientific investigation [2].  Inter-aural time delay (ITD), and intensity differences (IID), spectral envelope cues, and the "precedence effect", all play their part in recognizing the location of sources when room reflections are present. In addition to affecting localization: "The lagging sounds can contribute markedly to perceived timbre and to the sense of a room's acoustics. If the acoustical features of the lagging sounds are consistent with them being echoes of the leading sound, then the lagging sounds are perceptually suppressed, and are not heard as separate auditory events. On the other hand, if the lagging sounds cannot be plausibly interpreted as echoes, or if insufficient information is available to decide whether they are echoes or not, then they will tend to be heard as separate auditory events." [2]

Most of the research into audibility of reflections has been performed under anechoic conditions. Detection thresholds and phantom image shifts for a single reflection versus delay time and amplitude have been studied extensively. But, the results cannot necessarily be translated to binaural listening in reverberant spaces. Nevertheless, the prevailing school of thought amongst audiophiles holds all reflections to be detrimental. The prescription is to either absorb or diffuse reflections as much as possible. A room with only hard reflective surfaces and with no furnishings, having echoes and long reverberation time, is clearly not an ideal playback environment, but nor is an overstuffed room, full of absorbing materials and resembling an anechoic chamber. The ideal room may be a function of the type of speaker used. 
The typical, conventional box speaker has a power response that decreases over 10 dB from low to high frequencies, assuming a flat on-axis response. It is omni-directional up to a few hundred Hz and becomes ever more forward radiating with increasing frequency. An open baffle, dipole speaker has at least 5 dB less power response variation between low and high frequencies. While box speakers seem to perform best in relatively dead rooms, I have found that dipole speakers show their qualities better in a rather live room. 

In general, the more a room resembles an anechoic chamber, the less the off-axis radiation contributes to the sound at the listening place. The type of dipole speakers I have worked on, all try to duplicate the on-axis response for off-axis horizontal angles up to +/-60 degrees, albeit at decreasing levels and up to about 5 kHz. This has two effects. The level of the reflected sound is angle dependent and can be reduced by speaker orientation. The acoustic features of the reflected sound are consistent with the direct sound. Both of these help in suppressing a perception of timbre change and decrease a sense of the room's acoustics. Consequently, you hear more of the speaker and less of the room.

Much has been made about the wall behind an open baffle speaker, the front wall from the listeners perspective. The wall reflects the rearwards radiated sound which is 180 degrees out-of-phase with the forward radiated sound. The interference between the two leads to progressive cancellation at low frequencies and comb filtering at higher frequencies for steady-state sounds. A corresponding effect occurs with a box speaker, at least up to several hundred Hz, only the comb filter frequencies are different and the low frequencies are boosted. All of this is controlled by the distance between speaker and wall. If the speaker is at least 1 m (3 ft) in front of the wall, then the reflected sound is delayed by about 6 ms, which is sufficient not to be perceptually summed with the direct sound. The cognitive process disassociates it from the speaker and attributes it to the room with a sense of space.
Panel type open baffle speakers can have specific problems with the back wall. The radiation impedance seen by the panel goes to extreme values at frequencies where the distance is a multiple of a quarter wavelength. The motion amplitude of the very low mass panel may change due to the change in air load, causing a change in frequency response. Also, a large panel area creates a lobing polar response at higher frequencies and the reflection can become colored in timbre, shift angle and cause unstable imaging.

I have observed that a certain amount of lateral reflection is necessary for creating a believable illusion of the space in which the recording took place or to give a satisfyingly rich musical experience [3,4,5]. Flush (soffit) mounted speakers strike me as producing a sound that is two-dimensional, analytical, but ultimately lifeless. Highly directional horn speakers create a similar negative impression. In the tweeter range, wide horizontal dispersion removes harshness and adds airy-ness in my experience. This only makes sense if the reflected sound intensity has been increased. It might also explain why ribbon tweeters, which can be very narrow sources leading to wide dispersion, are so highly regarded, despite their poor vertical polar response. 

RT60 for my listening room is around 550 ms and pleasantly lively for conversation and socializing. I do not like a muffled cocktail lounge ambience. The two side walls are mostly double pane glass. The room is very long. A large brick fire place is 1.5 m (5 ft) behind the speakers. Imaging is very precise, given a good recording, with a smooth spread of the venue acoustics between the speakers and slightly beyond. The images are placed behind the plane of the speakers, never in front. The speakers disappear completely.

Sound diffraction at the edge of a box speaker baffle is claimed to degrade stereo imaging. I have not experienced this myself for speakers with typical flat baffles for drivers up to 8" (20 cm), nor have I seen scientific evidence. The low amplitude diffracted sound wave occurs so early after the direct sound (<500 us), that the precedence effect does not come into play and summing localization occurs [6].  What is significant, is that the "baffle step", the transition from 4p to 2p-space radiation, occurs at higher frequency for a narrower baffle. This keeps the power response more uniform over a wider frequency range and the spectral signature of any reflection more like the on-axis response. Large radius or wide chamfered edges smooth the high frequency response on-axis and off-axis.

The question remains: When is room treatment necessary and for what purpose? 
Specular reflections [7] can be diffused with quadratic residue diffusor panels, but this will not change the reverberant sound energy in the room. Diffusors become very large for frequencies below 500 Hz. Reflections can also be absorbed. This too works best above 500 Hz. Treatment for lower frequencies becomes exceedingly more space consuming and below 100 Hz requires custom solutions. 

[1]  William M. Hartmann, "Auditory localization in rooms", Proc. AES 12th International Conf., Copenhagen, 1993, "The perception of reproduced sound", pp. 34-39 
[2]  Brian C.J. Moore, "Controversies and mysteries in spatial hearing", Proc. AES 16th International Conf., Rovaniemi, 1999, "Spatial sound reproduction", pp. 249-258
[3]  Arthur H. Benade, "From instrument to ear in a room: Direct or via recording", JAES, vol. 33, no. 4, 1985 April
[4]  David Moulton, "The creation of musical sounds for playback through loudspeakers", Proc. AES 8th International Conf., Washington, 1990, "The sound of audio", p. 167
[5]  https://www.sawonline.com/wp_mix_article1.html
[6] Jens Blauert, "Spatial hearing. The psychophysics of human sound localization", MIT Press, 1997
[7]  James A. S. Angus, "The effects of specular versus diffuse reflections on the frequency response at the listener", JAES, vol. 39, no. 3, 2001 March 
Top

E - Line sources

As a line source I define a speaker with a very narrow and long radiating surface, which is used at frequencies above  f = c/2L, where its length L is greater than 1/2 wavelengths. Often such line source consists of a vertical array of a large number of identical drivers on a narrow baffle. In other cases it might be a ribbon like, narrow and long conductor in a magnetic field, or a narrow and tall electrostatic source. Specifically, I am referring to lines that are at least 1.5 m (5ft) long.

Under free-field conditions such lines will have a wide and fairly uniform  horizontal radiation pattern The vertical polar response will be very irregular and highly distance dependent, because of the finite length of the source [1]. In a room, though, the images of the source in floor and ceiling will make it appear as infinitely long, the more so, the less the gaps between the ends of the source and the reflecting floor and ceiling surfaces. Consequently, the line launches a cylindrical wave and illuminates the room very uniformly. The acoustics of the room, therefore, are of similar importance as for an omni-directional point source. At sufficient distance the direct SPL falls off with the square root of distance, which improves the direct-to-reverberant sound ratio over that of a point source.  

It surprises me that in a reverberant environment, such as a typical living room, these radiators give little indication of poor vertical polar behavior, even when the line does not fully extend to floor and ceiling. Clearly, the reverberant field in the room fills in any perceptual notches in the polar response. Standing close to the line and moving with one's ears up and down, the acoustic image does not change and follows the ear over the active length of the line, almost to its ends. Imaging is very precise, when standing or seated in the near-field of the line, which is closer than the typical listening distance given by the apex of an equilateral triangle. At greater distances the images grow in height. This can be impressive on big orchestral pieces, as if looking through a large window onto the sound stage. Small acoustic sources, like a vocalist or a cello, though, become overblown in size. Line source speakers are at their best when listened to close-up like giant headphones. This preserves proper size imaging and gives the illusion of a large sound stage. 

Vertical height of the sound stage adds to its realism. I find small 2-way speakers very unsatisfactory in this respect, even when they are correctly positioned at ear level height. Line sources provide this height, as do single tweeter symmetric driver layouts like LM-UM-T-UM-LM. In the latter case separate frequency ranges are assigned to each symmetrical set of drivers LM-LM and UM-UM, such that any free-space vertical lobing is minimized. This type of source suffers considerably less from the image size distortion of a uniform line.

An added advantage of tall sources is their relatively large radiating surface area, which means small excursions and, therefore, progressively lower non-linear distortion for a given maximum sound level. The low distortion aspect is even more important, than sound stage height, wide angle horizontal dispersion and uniform power response. Low distortion means increased dynamics and clarity, and the ability to play back at near live levels without listener strain. It brings the naturally occurring distortion mechanisms of the ear into play, which are necessary elements for creating an illusion of reality. 
Sensitivity can be high. A line with 25 identical drivers connected in series-parallel to obtain the same combined impedance as that of a single driver, will have 14 dB higher SPL for the same voltage applied. 
Stored energy is not necessarily low because of the many cabinet and driver chassis cavities.

I would encourage more investigation of line sources for use in very large venues, as for public address or entertainment speakers. For typical domestic environments they are unnecessarily large. Today, the same low distortion sound pressure levels can be obtained from far fewer drivers and without the image distortion. 

[1]  Stanley P. Lipshitz, John Vanderkooy, "The acoustic radiation of line sources of finite length", 81st AES Convention, Los Angeles, 1986, Preprint 2417
Top

F - Group delay and transient response

Few studies have been made about the audibility of linear distortion of the time waveform for an acoustic signal. Some people have great expectations about the improvements that DSP technology can bring to loudspeakers and crossovers through linearizing their phase response, which should result in a perfect transient response. The step response fidelity is often taken as a measure of performance. The step response is the integral of the impulse response over time, and belongs to a frequency response, that has been rolled off at 6 dB/oct towards high frequencies, starting from zero frequency.

It is instructive to review the step response of a practical 3-way speaker and to compare it to a full-range speaker. Both speakers are assumed to have a flat frequency response that is 3 dB down at 20 Hz and 20 kHz, rolling off at 12 dB/oct like a filter with Q = 0.7. The 3-way speaker has LR4 crossovers at 100 Hz and 2 kHz as in graph (a).

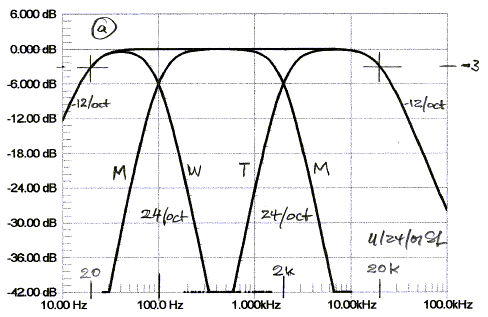
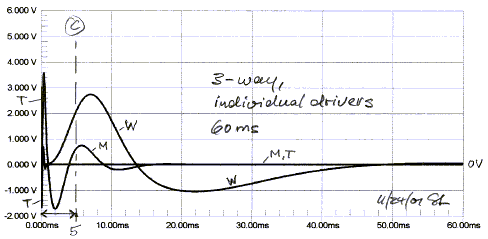
The step response of the full-range speaker FR is far from perfect, because the loudspeaker is a bandpass and in this view (b), over a 60 ms time window, the low frequency highpass dominates the shape of the curve. At time Tx the response crosses the zero axis. The positive area under the curve between zero and Tx equals the negative area between Tx and very large time, because a bandpass system cannot pass dc. Equivalently, a speaker cannot set up a permanent pressure increase, as would be required for perfect step response.

DSP based equalization can move Tx out in time, but how far is enough needs to be established. The aural integration time window is about 50 ms, before echoes are noted. 
The step response of the 3-way looks even further removed from the ideal and it is easy to understand why people like to think this should be accompanied by audible defects. The response is the sum of woofer, midrange and tweeter outputs (c). The tweeter response, barely visible on the 60 ms time scale, really shows up in a display of the first 5 ms (d).

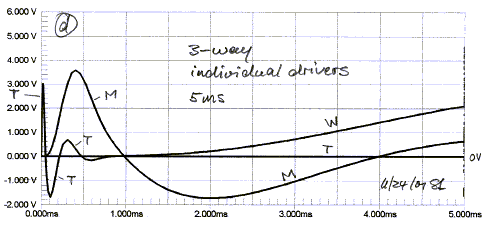
Note that the tweeter response has settled in 1 ms. The midrange has its second zero crossing at 4 ms. The woofer has barely started to move after 2 ms. A time window of 5 ms or less is frequently used in speaker reviews to comment about driver polarities and waveform preservation (e). This covers less than 10% of the transient response time for many speakers.

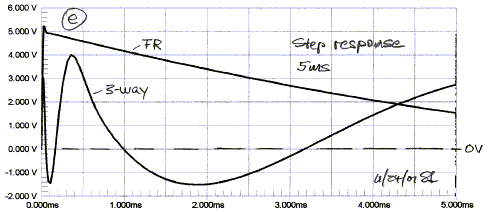
A recent audiophile magazine review of a 3-way speaker, with response similar to (e) above claimed: "... The step response indicates that the tweeter and midrange unit are connected with positive acoustic polarity (the sharp up/down spike at 0 ms and the lazier triangle 0.4 ms later), while the woofer is connected with negative polarity (the broad, negative-going hump centered between 1.5 and 2.5 ms). The actual drive-unit polarity doesn't matter except when it comes to waveform preservation -- and the jury is out on that subject."
More understanding of the subject matter is obviously needed.

The phase of a speaker's frequency response has to change linearly with frequency in order to preserve waveform fidelity. This is equivalent to saying, the group delay has to be constant (f).

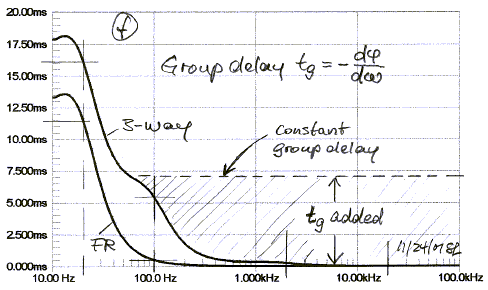
Group delay increases dramatically at low frequencies, even for the full-range speaker. To equalize the 3-way speaker down to 50 Hz a great amount of delay would have to be added to the higher frequency portion of the spectrum. This is practically impossible to accomplish with analog means and not completely trivial with DSP either. 

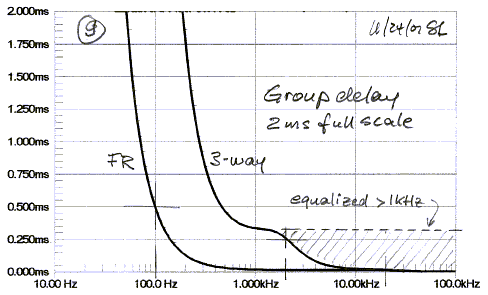
If delay equalization is restricted to higher frequencies, where digital processing becomes easier because of shorter time record lengths, then large portions of the spectrum remain distorted (g). Such solution may improve the first few milliseconds of the step response, but it remains to be shown unambiguously that if this is audibly noticeable. It will enable fairly accurate reproduction of square-waves with frequencies above 1 kHz though.

With similar effort a 2-way speaker could be delay equalized over a wider frequency range. A 2-way loudspeaker is either marginal in frequency coverage or in dynamic range and improvements in its transient response might be easily masked by other shortcomings. A full-range or single driver speaker is even more limited and not a contender, except in the form of headphones. 

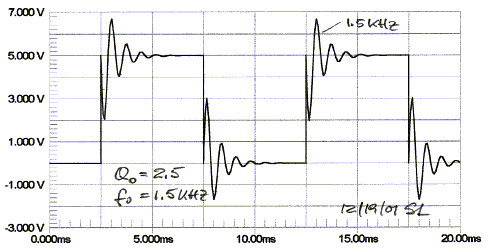
I have used headphones to convince myself that the phase distortion introduced by LR4 crossovers around 2 kHz and at 100 Hz are not audible. For example, a 1.5 kHz LR4 crossover with 24 dB/oct slopes forms a second order allpass filter with Q0 = 0.7 for its pole and zero locations. 

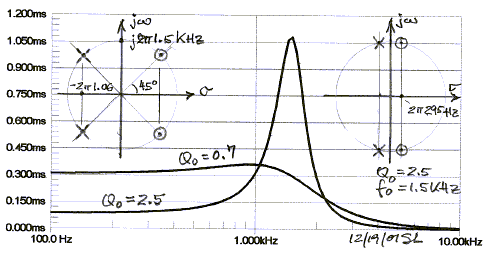
Its non-linear phase shift and non-constant group delay in the crossover region cause waveform distortion which is not audible, though.

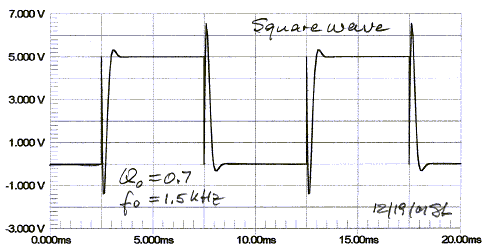
If the allpass Q0 is significantly higher, like Q0 = 2.5, then the phase distortion does become audible. In the case of the 100 Hz square-wave a high pitched sound is added. The frequency response, though, is completely flat.

 I am not certain what happens in the range below 100 Hz and I have strong suspicions that this is the region where delay distortion is audible. It is also the region where delay really accumulates via vented and bandpass woofers, and the great numbers of dc blocking capacitors in the signal chain from microphone to speaker terminal.

Further experiments might be performed with a high quality 3-way speaker system, whose phase response or group delay have been carefully measured or modeled. Knowing the phase distortion, which the speaker will introduce, use digital signal processing with the appropriate software to pre-distort various music files on a PC, such that the overall playback phase response will be linearized. Burn the newly created files to CD-R. For comparison take the same music files and add a constant delay to them, so that pre-distorted and un-distorted material have undergone the same process and any artifacts are common to both. In this way one could switch directly between nearly identical tracks of a CD-R and listen for differences that can only be the result of phase response differences.

Further thougths ...

Bohdan Raczynski compiled "Some Attributes of Linear-Phase Loudspeakers", which points to the mechanisms by which we recognize the direction to a source and its size (Fig. 3). 

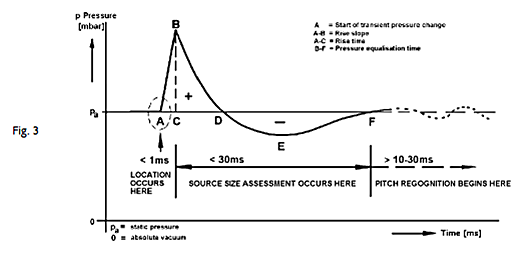
Compare the time scale to Figures (b) and (e) above for a 3-way loudspeaker to note to which degree it distorts the wave form in the location determining and size assessment time frames. Is that audibly significant? 
It makes sense to me that bass from a linear-phase speaker sounds different. Group delay at the low end does seem to matter. Vented versus sealed box bass certainly sounds different. Transients in the range above 2 kHz, in the ILD range of hearing, are used for location determination, but also signal arrival time differences in the ITD range below 800 Hz. The stereo phantom scene, the mental illusion derived from cues in left and right loudspeaker signals and often masked by the room's response to them, could therefore be affected in terms of its spatial aspects and clarity, but less in terms of timbre. The high degree of spatial distortion in headphone rendering would invalidate linear-phase listening tests using them. I suspect that "hearing absolute polarity" is a low frequency phenomenon, differentiating between the polarity of the doublet wave form during rapid compression/rarification and its opposite polarity during much slower relaxation. I doubt that one can hear the polarity of a high frequency "click". But I have been told that it can sound like "clack" when phase distorted.
Today it is not that impractical anymore to linearize the overall phase response of a speaker using DSP and to hear the results. As with all listening the experience is subjective and its validity can only be confirmed by comparison to the sound of real sources. 

Victor Staggs wrote to me about group delay distortion effects at the low end of a loudspeaker's frequency response:
"... I only claim that the group delay is audible for signals containing transients with sufficient energy at low frequencies. I wouldn’t expect to hear the effects of group delay in pipe organ music, for instance." 
   I agree. 
Top

G - Multitone distortion

The measurement of harmonic distortion gives an incomplete description of the non-linear behavior of a loudspeaker, even when the exciting sinewave is swept to generate a continuous display of distortion products. Still, it is very useful data for comparing devices. 

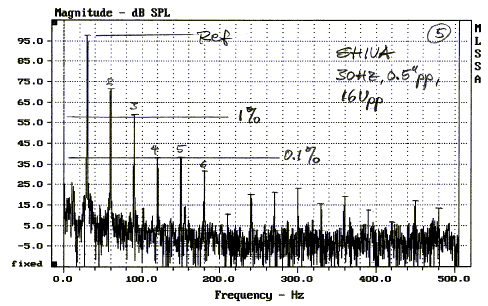
More information is obtained when two tones are used, because now also the various sum and difference products of the two stimuli and their harmonics can be measured with a spectrum analyzer. These intermodulation products are usually higher in amplitude than the related harmonic distortion products. I cannot emphasize enough, that anytime when a device introduces harmonic distortion, it will generate intermodulation distortion, when more than one tone is involved. Some seem to think that a little bit of 2nd harmonic distortion, as often generated by tube equipment, has a pleasing effect, because it enriches the natural even harmonics of acoustic instruments. It will also generate non-harmonic intermodulation, and has little to do with accurate sound reproduction or even generating the illusion of a real sound. Instead it imparts a euphonic sameness to all sounds.

Real sounds contain many tones. A woofer might be tested with a set of 5 tones that are not harmonically related, such as 20, 28, 44, 64, and 92 Hz, but which cover the woofer's intended operating range. Each of the tones is a multiple of 4 Hz, with factors 5, 7, 11, 16, 23, and the resulting distortion products will occur at 4 Hz intervals.

The richer distortion spectrum makes comparison between devices easier than relying on the few data points that harmonic distortion provides. The time waveform for the sum of the 5 tones looks very erratic but is, of course, periodic. A very simple looking, but highly effective test signal is a sinewave that is 100% amplitude modulated at 1/10th its frequency. This type of stimulus is especially useful for stressing vibrating systems and audibly detecting the onset of distortion as its amplitude is increased. To avoid permanent damage only bursts of this signal should be used.

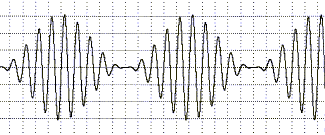
The undistorted spectrum consists of the carrier b and two sidebands a, c, which are 6 dB down and offset by b/10. The potential distortion spectrum contains harmonic and intermodulation products. A few of those are shown below. They occur at intervals b/10.

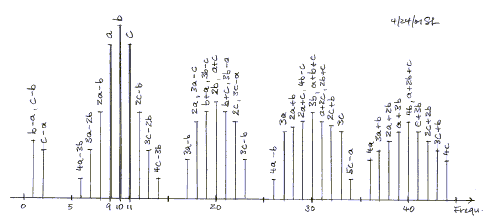
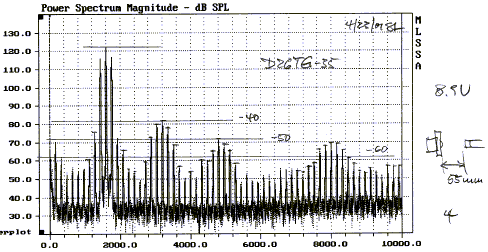
The close-up measurement of a tweeter reveals the dense sound spectrum generated by the cluster of 3 tones at 1.6 kHz.

I have found these and other types of multitone test signals useful for sorting and relative ranking of drivers. Their subjective evaluation when part of a complete speaker correlates closely with the measurements. It should be understood that distortion has audible effects long before it is perceived as distortion. For example, it might enhance sonic detail, analogous to contrast enhancement in a photo. 

I think a recent experience while attending a San Francisco Symphony performance gave me further insight into why we are able to tell a loudspeaker from a live instrument. Loudspeakers reproduced pre-recorded material, interspersed with the musicians' playing, during the performance of several modern pieces of music. It seemed, that coming out of the loudspeaker a sound carried a comet like trail of newly created sounds with it, that formed a continuum of background sounds, which was completely absent from the live instruments and made the speaker immediately recognizable as such. Live instruments had a space between tones, like a black background, and even en mass always remained articulate. I believe the observation had nothing to do with recorded ambience, which was present occasionally, or with hall reverberance. It reminded me of other incidences, where I had easily recognized live instruments, though there was no reflection free path between the source and my ears, and where I then struggled to describe to myself what would have been different, if a loudspeaker was playing instead. It seems to me that the ongoing-ness of sound is one of the major problems with speakers. It shows less with recordings of a single voice with small accompaniment, so often favored by audiophiles, but in complex passages of classical orchestral music and choral works.

Much work remains to be done before firm predictions of sound character can be made from distortion measurements. Wolfgang Klippel has developed a practical methodology for characterizing and auralizing the effects of various distortion parameters in woofers at large excursions. Researchers at Cerwin Vega have done extensive theoretical work [1]. They concluded amongst other things: "... With an increase in the number of tones, the multitone provides increasingly detailed information about the nonlinear behavior of a DUT. An ultimate increase in the number of tones leads to a noise-like signal that may hypothetically provide complete information about a nonlinearity-impaired DUT, but demands an enormous amount of calculation, if a high-order nonlinearity is to be assessed."

[1]  Gene Czerwinski, Alexander Voishvillo, Sergei Alexandrov, Alexander Terekhov, "Multitone testing of sound system components - Some results and conclusions, Part 1: History and theory", JAES, Vol. 49, No. 11, 2001 November, pp. 1011-1048. 
"Part 2: Modeling and Application", JAES, Vol. 49, No. 12, 2001 December, pp. 1181-1192.
Top

H - Suggestions and hearing

It is common practice in promoting audio products to tell a customer what  to pay attention to in what he is going to hear, then to follow that with a demonstration, and afterwards to tell him again what he heard. It is the same process, whether it is a manufacturer's representative speaking with a store sales person, or the sales person talking to a prospective buyer. It is almost impossible for the target person not to hear, what has been suggested they will hear. 

The power of suggestion has been used to perform major surgery without anesthesia, where the patient experiences no pain. It has been shown with CAT scans of the brain that in such cases an information disconnect occurs between the region that responds to pain, and the region of the brain that gives the patient the perception of pain. Thus, the normal perception process has been altered through the verbal, non-physical preparation that  the patient received beforehand.

The influence of suggestion, especially when frequently repeated, should not be underestimated in the field of hearing. Many listeners have not acquired an auditory reference that is based on un-amplified sounds and are thus easily misled in matters of accuracy. Double-blind and ABX product comparison tests are frowned upon in some audio circles, though they remove any influence of suggestion, self or other generated. 

I have no problem, if people enjoy their music more, because it has passed through a certain interconnect, has been transformer balanced, amplified without feedback, processed through a reissued WW2 transmitter tube and sent to their speakers on litz wires of specific arrangement and purity, except that the money for these suggestions could have been spent on better speakers, for real improvements in accuracy of dynamic range and resolution. After all, the loudspeakers are by far the weakest link in the chain of components that are needed for sound reproduction.

Addendum, February 2008:
If a tree falls in the forest, does it make any sound? No, except when a person is nearby that interprets the change in air particle movement at his/her ear drums as sound coming from a falling tree. Perception takes place in the brain in response to changing electrical stimuli coming from the inner ears. Patterns are matched in the brain. If the person has never heard or seen a tree falling, they are not likely to identify the sound. There is no memory to compare the electrical stimuli to. 

This apparently also works the other way around. If brain connections are set up in response to suggestions or expectations, then those will also be used to interpret incoming electrical stimuli. I am convinced that my friend who claims he hears the difference when he first demagnetizes a CD before playing it, he actually perceives it as such and it is reality for him. I cannot hear it, but then I have a low expectation of hearing any effect. It does not make sense to me that there could be a difference. 

This is a tricky area for a loudspeaker designer. I therefore try to focus on the basics of acoustic and electrical design and to stay out of it. I try to maintain firm auditory references by refreshing them periodically, like having season tickets for the symphony. It is easy to get lost. High-end audio is full of gadgets and supporting theories. For some this is the way to Nirvana. I prefer composers and performing musicians to lead me there.

By all means read: "This Is Your Brain on Music" by Daniel J. Levitin
Top

I - Digital crossovers

Some people think that digital crossovers will replace analog ones, because digital filters can be designed with desirable characteristics that are impossible to realize with analog circuitry. In particular, lowpass and highpass filters with extremely steep slopes and linear phase shift are possible. Steep slopes reduce the overlap region between drivers. Linear phase shift eliminates waveform distortion and merely causes a delay of the signal.  Such characteristics can be obtained from the digital equivalent of tapped delay line filters, which have a finite impulse response (FIR) duration that depends upon the number of taps used. Digital FIR filters can have almost any desired frequency response, if the number of weighted taps is made sufficiently high. [1]

The linear phase shift comes at a price. The impulse response rings. The more so, the steeper the filter slopes. Both lowpass and highpass sections of the crossover ring, but when the outputs are combined, as for a crossover, then the two impulse responses add to a non-ringing, delayed pulse.

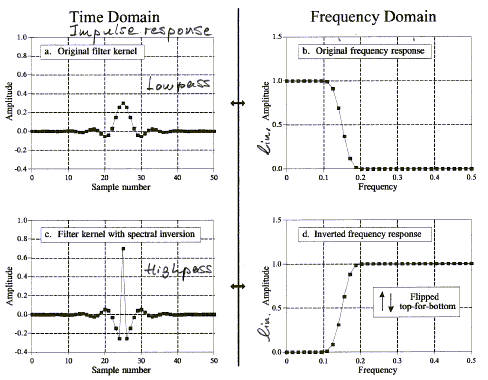
All would be fine, if we listened only in anechoic spaces or to speakers with coincident drivers. In reality we use speakers in rooms with reflections and reverberation and the the drivers are separated from each other due to their sizes. As a consequence the off-axis response of the speaker matters and contributes to what we hear. With the drivers non-coincident, the lowpass and highpass outputs are delayed different amounts at points off-axis, and the ringing is no longer canceled in the addition. In the best case the drivers might be coaxial, but this has another set of problems. Very steep crossovers can also cause a very abrupt change in the polar pattern of the speaker, when transitioning from a large diameter driver to a small one. Under reverberant conditions and/or listening off-axis this may have audible consequences.

A different type of digital filter has an infinite impulse response (IIR), from using recursive or feedback topologies. IIR filters can closely simulate all the known analog filter functions, but also have their associated non-linear phase shifts. The frequency response of a given IIR filter, such as a biquad, can be easily changed by reprogramming its coefficients. They are readily adapted to different needs. 

Digital filters have high accuracy and are very repeatable. They require high performance ADC, DAC and DSP.  Analog anti-aliasing and reconstruction filters are part of their design. Dynamic range, clipping and noise must be dealt with in the digital domain of numbers. In particular, the effects of numeric round-off upon filter shapes and distortion must be analyzed. For reasons of limited linear digital range the volume control must be located after the DSP to maximize bit resolution.  Non-linear distortion in all its forms between input and analog output has to be considered and minimized.  Loudspeaker drivers have their own inherent acoustic highpass amplitude and phase response, which must be accounted for in the digital crossover filter design. Electromagnetic compatibility (EMC) should be designed in and tested for.

[1]  Steven W. Smith, "The Scientist and Engineer's Guide to Digital Signal Processing"  
Top

J - Doppler distortion

Drivers are now available that generate very low intermodulation distortion, because their motor behavior is very linear. This may bring Doppler distortion to the foreground under certain conditions. Doppler distortion occurs when two tones of largely different frequency are radiated from the same membrane. It is a linear form of distortion, at least to the first order, which means its magnitude is independent of the amplitude of the higher frequency tone and distortion increases linearly with the amplitude of the lower frequency tone..

For example, a 18 cm (6.5 inch) driver with 126 cm² cone area like the Seas W18E001 may radiate a 50 Hz low frequency tone at 5 mm peak excursion, which produces 86 dB SPL at 1 m. The sinusoidal cone displacement has a peak velocity of:

V_p = 2 p f_L X_p = 1.57 m/s

A 2000 Hz tone which is radiated simultaneously will have its wavelength shortened and lengthened at a 50 Hz rate as the cone velocity is added to and subtracted from the speed of the sound's propagation (c = 343 m/s). Based on a relatively simple analysis this results in a frequency modulation of the 2000 Hz tone with a peak deviation of:

Df_H = 2 p f_L f_H X_p / c = 9.2 Hz

The modulation distortion can be reduced to 5.5 Hz for the same 50 Hz SPL by using a W22EX001 driver with 220 cm² cone area.

1. the FM threshold of hearing, 
2. masking by the low frequency tone,
3. FM to AM conversion due to room resonance.

The FM threshold is typically determined at a low modulation rate of 4 Hz and is about 0.35% for frequencies above 500 Hz, corresponding to 7 Hz deviation at 2000 Hz. Higher modulation rates around 70 Hz may be perceived as roughness of the tone.
Masking is dependent upon the relative levels and frequency separation of the two tones.
A room with a reverberation time T₆₀ = 500 ms has resonances with a 3 dB bandwidth of BW = 2.2 / T₆₀ = 4.4 Hz. The slope of the filter curves is about 3 dB per BW/2 or 1.4 dB/Hz. Thus, a 9.2 Hz deviation of the 2000 Hz tone could generate up to 12.9 dB change in its amplitude, if the rate was slow enough so that the sidebands of the FM signal are not significantly attenuated by the filter.

Doppler distortion of a driver can be measured with a spectrum analyzer by observing the amplitude of the frequency or phase modulation sidebands A_s relative to the carrier A_H.  The relative amplitude for the first pair of sidebands is approximately

A_s / A_H = 0.5 Df_H / f_L = p f_H X_p / c = 0.09 

or 20.7 dB below the 2000 Hz carrier A_H, regardless of f_L as long as X_p = 5 mm. This corresponds to a phase modulation with a maximum phase deviation of  2 A_s / A_H = 2 x 0.09 rad = 0.18 x 180 / p = 10.3 degree.
The sidebands occur at the same frequencies f_H - f_L and f_H + f_L as the 2nd order intermodulation products of the two tones. They can be differentiated by changing the applied signal levels. Doppler sidebands decrease 3 dB for a 3 dB reduction in signal levels, whereas 2nd order intermodulation sidebands decrease 6 dB. The two sidebands may be of different amplitude when both forms of distortion are present, because the intermodulation products are in-phase with each other, the FM sidebands are out-of-phase. This fact allows a decomposition of unequal sidebands into their two constituent odd and even parts. 

I have experimentally verified the results of the above analysis by measuring the relative amplitude of the sidebands in the frequency spectrum. The Doppler effect is very difficult to measure in the time domain, though, because of the small amount of phase shift (e.g. 10.3 degree) that it causes for the higher frequency tone. Rod Elliott claims to have been able to measure the shift in the time waveform. An analysis of his observation confirms that the maximum phase shift occurs when the cone is at its maximum excursion, where its velocity is zero. The Doppler analysis usually mentions only the maximum frequency shift, which occurs when the cone has its highest velocity, or when going through zero excursion for a sinusoidal low frequency tone. Both results are correct and are a consequence of the fact that the radian frequency w = d(phase) / dt . This leads to a 90 degree phase shift between time varying frequency and phase for a sinusoidal signal. Either way of looking at the situation results in identical magnitudes of the spectral components. It all is the Doppler effect, though I have not seen the associated phase modulation pointed out in text books.
Top

K - Room response equalization

In theory, digital signal processing allows to remove completely the effect of the room upon the frequency response of a loudspeaker at the listening position, but only over a very small region in space. The principle is illustrated by correcting the output signal from a loudspeaker in order to cancel the effect of the inevitable floor reflection upon the response at the listener's place.

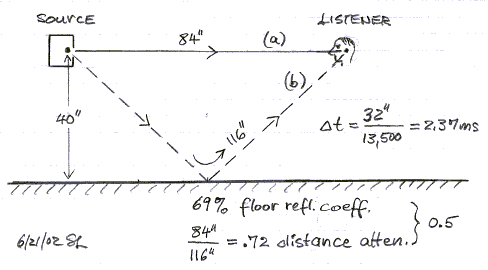
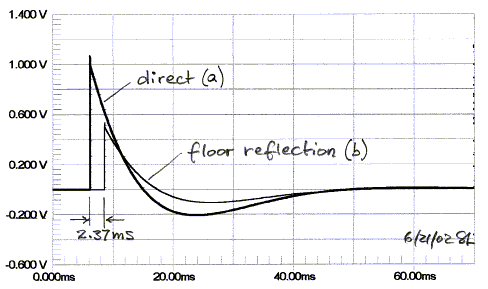
The model assumes a speaker with 20 Hz to 20 kHz response. The floor is not perfectly reflecting and together with the attenuation, due to a 32" (0.81 m) longer path length for the reflected signal (b) than the direct signal (a), the amplitude of the reflected signal at the listener will be half that of the direct signal. The floor reflection is delayed by 2.37 ms. The step  response at the listener position is the sum of direct and reflected signals (c).

The frequency response at the listener position shows the typical comb filter behavior (e). At low frequencies the floor reflection adds to the direct sound (d). The first minimum in the response occurs when the 32" path length difference corresponds to 1/2 wavelength and causes 180 degrees of phase shift, which is at 211 Hz. The next peak is at 422 Hz, followed by a minimum at 633 Hz, and so on. The response is non-minimum phase and can be corrected with a minimum phase parametric equalizer to some degree, but only at the very low frequencies.

The effect of the floor reflection can be cancelled by pre-distorting the direct signal from the speaker. After 2.37 ms from the start the direct signal has to have a negative step to cancel the first reflected signal which arrives at the listener. The negative step in turn causes a floor reflection which must be cancelled by a positive step 4.74 ms later, and so on. Since the floor reflection is attenuated, consecutive correction steps will decrease in amplitude (f). 

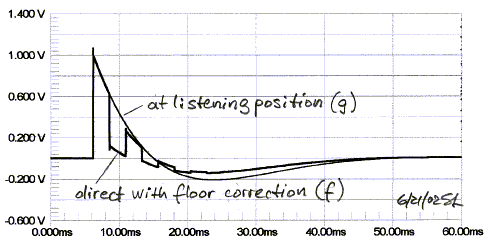
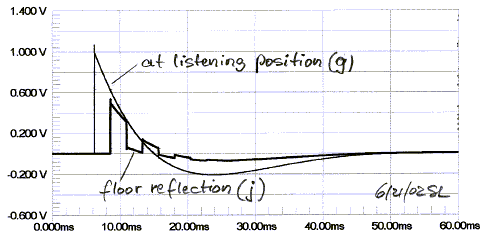
Direct (f) and reflected (j) signals combine at the listener position for a perfect step response (g), identical to the anechoic response (a).

The frequency response of the pre-distorted direct signal (h) may have large peaks to correct for the notches in the room response (e). 

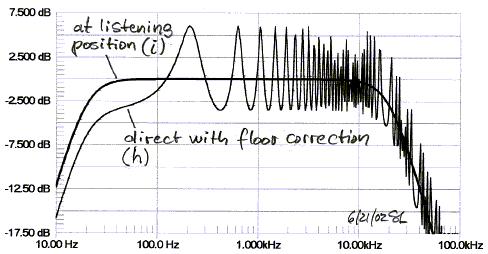
It should be obvious that this form of room correction is a delicate balancing act, particularly to maintain equalization at higher frequencies.  Small deviations in listener position from the point for which the speaker was equalized, cause large changes in room response. In real rooms there is not only the floor reflection, but all the many other reflections from walls, ceiling, furniture, etc. that enter the correction of the speaker. Since the correction can at best be valid only for a single sweet spot, it will necessarily send a distorted signal to every place else. Even at the sweet spot the system performance does not degrade gracefully, when the correction is less than perfect. Gross spectral distortion is introduced at higher frequencies and may become aurally distractive.

Room equalization with DSP will require a new understanding of what should be corrected, and what should be left alone, to meet a specific overall objective for sound reproduction. For example, the comb filter response due to floor reflection is intimately familiar to us, since most sounds we hear, have at least a floor reflection. As we grew up and became taller the comb spectrum shifted towards lower frequencies. How do we use the information conveyed by it? How important is it to correct for it in a speaker setup? The issue with room equalization is not about sufficient computing horse power, but how and where to apply it. 

The following two references are highly recommended reading before embarking on any digital room equalization project.

[1] Louis D. Fielder, Practical Limits for Room Equalization, 111th AES Convention, 2001, Preprint 5481
[2] Scott D. Snyder, Active Noise Control Primer, Springer/AIP, 2000
Top

L -  H-frame and U-frame open-baffle woofers   

The analysis of a H-frame dipole woofer is rather simple due to the symmetry of the mechanical structure combined with the fact that front and rear acoustic outputs are of reversed polarity. The influence of a number of important parameters upon the radiated field can be ignored, since their effect is equal in magnitude on both sides of the baffle and cancels because of the 180 degree phase difference. The analysis becomes more complicated when the frame is no longer symmetrical and the driver is moved closer to one of the openings of the frame.

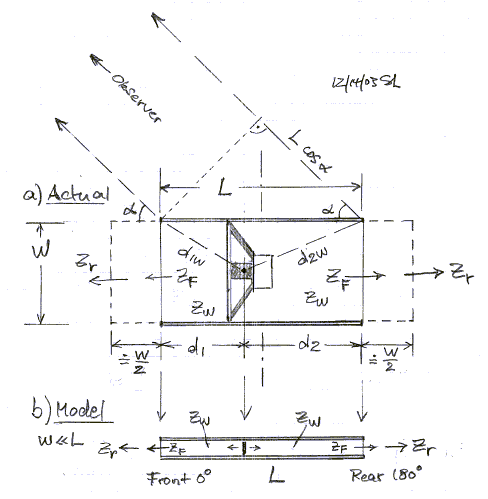
The baffle structure has a physical length L = d1 + d2 and width and height W. The acoustic length is larger by about W/2 on each end, similar to the end correction of the vent of a Helmholtz resonator. For determining the polar pattern I will assume the case where W << L, and work only with the physical length L. Any calculated 1/4 wavelength resonance frequency will be higher than in actuality, but that does not invalidate the relative performance at different driver positions d1.  The observation point is far enough away so that the outputs from front and rear are seen at the same angle a. The two propagation paths then differ in length by Lcos(a).
I assume that the driver is operated in its mass controlled region and acts as a volume source or like an electrical current generator. Using an actual driver model would complicate the analysis and only be beneficial if we needed to know absolute numbers. I will also assume that the source is fully symmetrical, as would be the case with two drivers having their magnets pointed in opposite directions. A single driver would introduce additional phase shift on its backside due to the acoustic elements associated with the cavities around the basket and magnet structure. 

Looking from the driver cone towards the rear of the baffle the displaced volume propagates through a waveguide of acoustic impedance Z_w. If the waveguide were closed off at its end by a wall, then the volume flow would come to a stop as it reaches the wall and be reflected back to the source. The wall can be described by an infinite flow impedance Z_F. When the the waveguide is open ended the corresponding flow impedance Z_F is nearly zero. In reality, Z_F is an acoustic mass or, electrically, an inductive element. A small percentage of the flow is reflected back from the opening towards the source. When the wave hits the cone it is reflected back towards the opening, where again a small fraction of it bounces back to the cone. This back and forth travel sets up a resonance at the frequency where d2 equals a quarter wavelength. The major percentage of the volume flow exits the opening and expands into the larger free space. Consequently, its pressure drops relative to what it was inside the waveguide. This can be described by an impedance Z_r which is less than Z_w. The impedance mismatch exists at all frequencies. The pressure developed across the real part R_r of this radiation impedance Z_r is the quantity of interest.  

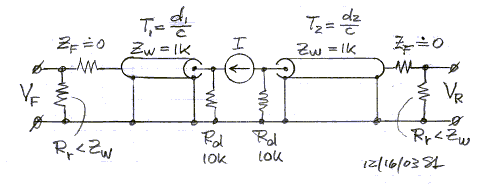
The circuit above describes the acoustic behavior in electrical terms. The two loss-less transmission lines are specified by their impedance Z_w and propagation delay T as function of their length d. The current generator has a finite impedance R_d >> Z_w. The flow impedance Z_F is assumed to be zero for the moment. The voltages V_F and V_R across the radiation resistors R_r are summed vectorially using the path length difference L cos(a) to determine the phase shift between front and rear outputs. The summed signal is proportional to the SPL at observation angle a.

It is important to work with representative values of R_r in the electrical model. Assuming R_r = 400 and Z_w = 1000 leads to a 1/4 wave resonance peak of about 6 dB. This is in the range of peak magnitudes that I have measured in the opening plane of H-frame woofers. All modeling is done for L = 0.6 m (24"), thus for the symmetrical H-frame the resonance occurs at 340m/s / (4 x 0.3m) = 283 Hz. The graph below shows magnitude and phase of front and rear radiation.

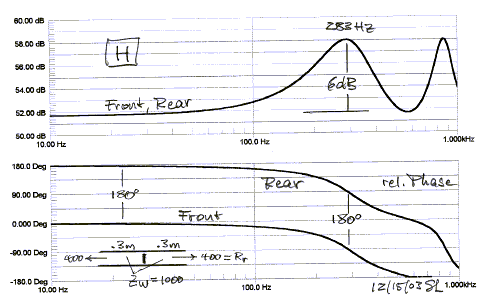
The frequency response at different observation angles a is obtained by adding the front and rear outputs vectorially. The analysis was carried out using CircuitMaker which is a SPICE program. Notice the slight amount of peaking near the resonance. The outputs sum to zero at 90 degrees. This would be off the graph. Instead, the 100 degree response is shown.

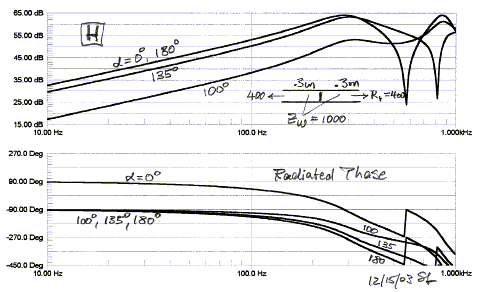
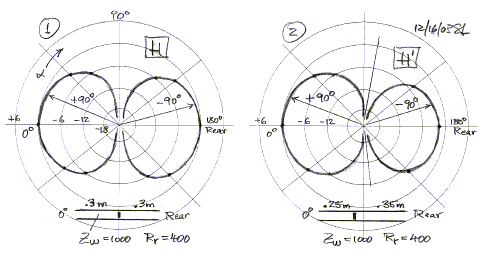
The polar plot (1) below is drawn on a dB scale with 6 dB increments between circles. Note that the phase of the acoustic wave to the left of a = 90 degrees is at +90 degrees, to the right at -90 degrees relative to the volume source excitation.
When the source is shifted slightly towards the front of the H-frame, then the null occurs at an angle a that is greater than 90 degrees. Also, the rear lobe decreases slightly in magnitude (2).

This trend continues and leads to the polar pattern (3) where the driver has been fully moved to the front of the waveguide. It is the U-frame configuration. The magnitude of the polar response has increased over that of the H-frame in the left half of the polar graph. On-axis and below 60 Hz by 3.4 dB, and at 90 degrees there is no longer any null. The right half of the graph takes on different shape that are quite frequency dependent. There is no null, but there is a small region where the phase of radiation is +90 degrees as compared to -90 degrees for the major portion of direction angles a. 

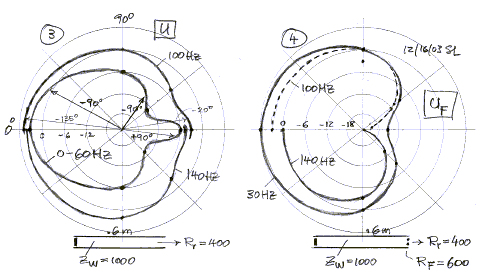
The shape of the polar pattern is strongly influenced by the impedance that the wave sees at the rear opening. By inserting a flow resistor R_F = 600, so that the waveguide is terminated reflection free, the response begins to look more like a cardioid. The on-axis response is 6 dB higher than that of the H-frame of same length L.

A cardioid polar response is obtained by the summation of a monopole response with magnitude 0.5 in all directions of radiation, M = 0.5, and a dipole with magnitude 0.5 on-axis, D = 0.5 cos(a), such that  C = 0.5 + 0.5 cos(a) . A monopole, such as a closed box woofer, is a pure pressure source. A dipole, like a H-frame woofer, is a pure velocity source. A cardioid or U-frame woofer would exhibit characteristics of both monopole and dipole. It is not clear what the room acoustic benefits might be. Unlike the H-frame with its 90 degree nulls, these types of mixed radiators can excite room modes at ALL angles. Even the null towards the rear of a pure cardioid woofer does not prevent the excitation of the room mode along the 0 degree to 180 degree axis. 

I have heard a rock band that used big Meyer Sound cardioid woofers in a very open setting. Walking around the stage there was a clearly audible reduction of low frequencies in the back. More slam for the audience, less for the neighborhood.
In a normal living room there could be an application for a cardioid above 1 kHz to reduce reflections from objects behind the speaker, if that were an issue.
It's a different story for microphones where the cardioid pattern can provide a clear benefit by reducing unwanted sound pickup.

If you have any interested in further analysis of the U-frame woofer, then go to  Appendix L .

Top

| Page 1 | Page 2 | Page 3 | Page 4 | Page 5 | Page 6 | Page 7 |